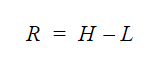How to Find the Range of a Data Set | Formula & Examples
In statistics, the range is the spread of your data from the lowest to the highest value in the distribution. It is a commonly used measure of variability.
Along with measures of central tendency, measures of variability give you descriptive statistics for summarizing your data set.
The range is calculated by subtracting the lowest value from the highest value. While a large range means high variability, a small range means low variability in a distribution.
Calculate the range
The formula to calculate the range is:
- R = range
- H = highest value
- L = lowest value
The range is the easiest measure of variability to calculate. To find the range, follow these steps:
- Order all values in your data set from low to high.
- Subtract the lowest value from the highest value.
This process is the same regardless of whether your values are positive or negative, or whole numbers or fractions.
| Participant | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
|---|---|---|---|---|---|---|---|---|
| Age | 37 | 19 | 31 | 29 | 21 | 26 | 33 | 36 |
First, order the values from low to high to identify the lowest value (L) and the highest value (H).
| Age | 19 | 21 | 26 | 29 | 31 | 33 | 36 | 37 |
|---|
Then subtract the lowest from the highest value.
R = H – L
R = 37 – 19 = 18
The range of our data set is 18 years.
How useful is the range?
The range generally gives you a good indicator of variability when you have a distribution without extreme values. When paired with measures of central tendency, the range can tell you about the span of the distribution.
But the range can be misleading when you have outliers in your data set. One extreme value in the data will give you a completely different range.
| Age | 19 | 21 | 26 | 29 | 31 | 33 | 36 | 61 |
|---|
Using the same calculation, we get a very different result this time:
R = H – L
R = 61 – 19 = 42
With an outlier, our range is now 42 years.
In the example above, the range indicates much more variability in the data than there actually is. Although we have a large range, most values are actually clustered around a clear middle.
Because only two numbers are used, the range is easily influenced by outliers. It can’t tell you about the shape of the distribution of values on its own.
To get a clear idea of your data’s variability, the range is best used in combination with other measures of variability like interquartile range and standard deviation.
Frequently asked questions about the range
- What is the range in statistics?
-
In statistics, the range is the spread of your data from the lowest to the highest value in the distribution. It is the simplest measure of variability.
- Can the range be a negative number?
-
No. Because the range formula subtracts the lowest number from the highest number, the range is always zero or a positive number.
- What are the 4 main measures of variability?
-
Variability is most commonly measured with the following descriptive statistics:
- Range: the difference between the highest and lowest values
- Interquartile range: the range of the middle half of a distribution
- Standard deviation: average distance from the mean
- Variance: average of squared distances from the mean
- What’s the difference between central tendency and variability?
-
While central tendency tells you where most of your data points lie, variability summarizes how far apart your points from each other.
Data sets can have the same central tendency but different levels of variability or vice versa. Together, they give you a complete picture of your data.
Sources in this article
We strongly encourage students to use sources in their work. You can cite our article (APA Style) or take a deep dive into the articles below.
This Scribbr articleBhandari, P. (September 25, 2020). How to Find the Range of a Data Set | Formula & Examples. Scribbr. Retrieved October 18, 2022, from https://www.scribbr.com/statistics/range/